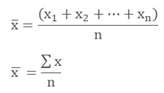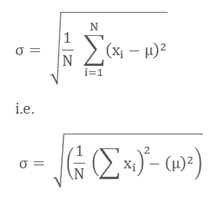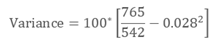Standard Deviation (S.D.)

1. What is Standard Deviation?

The quantitative degree by which every value in a given data varies from a Measure of Central Tendency is known as Standard Deviation (S.D.) It is denoted by Greek small letter σ. It is the positive square root of the Arithmetic mean of squares of deviations of a given data set from their Arithmetic mean.

The term ‘Standard Deviation’ was first used by Karl Pearson in 1894.

What is Measure of Central Tendency?

A single value which defines the properties of a given distribution is known as Measure of Central Tendency. Mean, mode and median are three main Measure of Central Tendency.  Mean is the most frequently used Measure of Central Tendency.

Why is mean used as a Measure of Central Tendency?

Mean is the sum of all the values of an observation divided by the total number of observations in a given data.

Formula of Arithmetic Mean

For the Frequency Distribution

Arithmetic Mean is used as a Measure of Central Tendency quite frequently because of the following merits:-

• It covers all the values of an observation.
• It allows further mathematical treatment.
• The error is minimized by using Arithmetic Mean for every value.
• Mean is the only value for which the sum of deviations for an observation is zero.

In case of Standard Deviation, Mean is represented by x-bar and in case of population observations mean is denoted as Greek letter mu (µ). Unlike Standard Deviation, the formula of mean remains the same in both the cases.

 

2. Formula for Standard Deviation

Population Standard Deviation

    

Sample Standard Deviation

Why are there two different methods of Standard Deviation S.D.?

S is an unbiased estimator for Population Standard Deviation. However, Sigma (σ) is a biased estimator for Population S.D. For large samples, the bias starts tending to zero as n increases. Hence, sigma is also considered as an equally important S.D.

For understanding the concept of Standard Deviation, the understanding of Variance is important

What is Variance?

Given a data set, the values vary about a measure of central tendency (mean, median, mode etc) and these measures are known as measures of variation or dispersion. Variance is one of the frequently used measures of dispersion where,

For Discrete Data:

3. How to Find Out Standard Deviation

In case of Population Values, procedural steps to find out Standard Deviation are as follows:

Step 1: Find µ.

Step 2: For each value, subtract the mean from the value and square it.

Step 3: Add all the values obtained through step 1.

Step 4: Divide the values obtained through 3 by total number of observations. (N in case of grouped data and ∑ frequencies in case of ungrouped data). We obtain the variance after this.

Step 5: Take the square root. This is S. D. of the observation.

 

4. Example of Standard Deviation

For Discrete Data, consider marks obtained in a class of 10 students (out of 50): 10, 20, 45, 45, 24, 8, 19, 45, 23, 35.

Here, N = 10
The mean of the given Data set is (10 + 20 + 45 + 45 + 24 + 8 + 19 + 45 + 23 + 35)/10 = 27.4

Here, N=10, variance= (3/N) = 182.4

S.D. = √variance → Standard Deviation = 13.49963

Here, S.D. is very high which indicates that there is a very high variation in marks of students in the class.

Given no. of people belonging to different age groups. Find the S.D. for grouped data?

Here, we take d = (x - 55)/10 now,

Mean = A + h* ∑fd/N where N= ∑f

Mean = 54.72 years.

{Variance is independent of change of origin but not of scale}  

→ 

→ σ = 11.88 years

5. Relationship of Standard Deviation with other Statistical Measures

Relationship between Standard Deviation and Variance

• Standard Deviation is positive square root of Variance.

Relationship between Standard Deviation and Precision

• Standard Deviation and precision are inversely related to each other.

 

 

6. Implications of Standard Deviation

Degree of variability of given observations. To understand it in a better way, consider three sets of observations given below:

1.

14

2

1

3

5

Mean = 5

Standard Deviation = 5.24404

Variance = 27.5

2.

5

4

6

4

6

Mean = 5
Standard Deviation = 1

Variance = 1

3.

5

5

5

5

5

Mean = 5

Standard Deviation = 0

Variance = 0

In these three observations, we noticed that the mean is 5 in all the three cases, whereas the Standard Deviation in 3^rd observation is 0 since there is no variation in values. In second observation, the Standard Deviation is very less since the values are closely related to each other and in first case, the Standard Deviation is very high due to very high degree of variation in the values.

 

 

7. Important Properties of Standard Deviation

• Standard Deviation is always positive.
• Standard Deviation of a constant is 0 i.e.  σ (c) = 0  where c is a constant.
• σ (X + c) = σ (X)
• σ (c * X) = |c|* σ (X)
• The above two properties show that Standard Deviation is independent of change of origin but not of change of scale.
• σ (X + Y) = √(variance(X) + variance(Y) + 2*covariance(X * Y)
• Standard Deviation is high when difference between values is large.
• Standard Deviation is nothing but sum of distance of values from mean divided by total number of observations.

 

 

8. Standard Deviation and Standard Normal Distribution  

Figure of normal distribution with mean µ

If X is  a random variable which is distributed normally such that X~N (µ, σ²) which has mean µ and σ S.D. then, the length within 2σ limits covers 68.26 of the area of the distribution. The area within 4 σ limits i.e. from µ - 2*σ to µ + 2*σ covers 95.46 area (% probability), the area between µ-3 *σ and µ+3 *σ is 99.7773 and rest of the area falls after µ±3σ.

 

 

9. Real Life Examples where Standard Deviation is used

• In finance, Standard Deviation is applied to the annual rate of return of an investment to measure the investment's volatility.
• Standard Deviation is used in models based on real life to check the variation in policies in real life situation in a much easier way.
• Standard Deviation is used to check hypothesis testing.
• Standard Deviation is used to find out confidence limits which is used to find out if there is  a significant deviation in quality of the products and the limit within which defects are permissible/tolerable limits and limits after which measures are to be taken.
• Standard Deviation is used to find correlation coefficient between two random variables.
• Standard Deviation is widely used in Normal Distribution.
• Standard Deviation is used to check p-values, t-test.

How to calculate Standard Deviation using excel or Scientific Calculators?

Excel and Scientific Calculators both have an option to calculate the population as well as the sample S.D.

Calculating of S.D. Using Excel

The command to find S.D. in excel is STDEVP (values) where values are chosen from a column.

Calculating of S.D. Using Casio Calcuator Fx 115-Es

• Click on the mode button.
• Press 3 for Stats.
• 1- for S.D. of single variable.
• Enter your data
• AC
• Shift +1 for Stats
• Select σ_x option.

 

 

10. Precaution that should be taken while using Standard Deviation

• It must be ascertained that which formula should be used for calculating Standard Deviation.
• 
  
• Visit: - www.justquestionanswer.com